Friday’s total lunar eclipse will be the longest of this century. What makes an eclipse (solar or lunar) long? I’ll answer that question below. But first, another topic of interest, the seasonal model of long waves, which is a particular adaptation of Kondratieff’s work. I became aware of the seasonal long wave model from the late P.Q. Wall in the early 1990s. In the late 1990s there was a particularly good listserv discussion group devoted to the Long Waves topic.
In the course of that group’s existence, I did research on eclipse cycles as drivers of the economic long wave. The result was my own model for the timing of the long wave. The particular eclipse cycle that returns this week was an integral part of that model, making now a good time to revisit that research. An extra bit of fun here is we get to revisit that model’s forecast originally compiled between 16 and 18 years ago.
Kondtratieff’s original work was identifying long-term cycles in wheat prices. The seasonal k-wave model adapted from that is shown below in its ideal form.
click chart to enlarge
The red line in the chart above shows the long-term trend in the price of “things.” The price of money (interest rates) is a good example to depict this cycle. The seasonal long wave model contends that equity prices (black) form a complete secular cycle within each half-cycle of the general price level (red.)
In the spring season, prices are rising. Inflation is increasing, but is contained. This climate is good for equities. Inflation and equities rise together.
In the summer season, inflation is now running hot. This climate is bad for equities. Equities now fall while price levels continue higher.
In the autumn long-wave season, prices have peaked and are now beginning to decline. This mild deflationary environment is once again good for equities. Interest rates are now declining while equities advance.
In long-wave winter, disinflation has given way to deflation. The price of money and the equities fall together.
The next chart shows how these seasons unfolded in the 20th century with long-term interest rates depicting the price cycle and Shiller’s 10 year CAPE P/E ration depicting equities. The chart effectively shows how equities complete two cycles for each single, long wave in prices.
click chart to enlarge
click chart to enlarge
The chart above shows the seasonal model as a ‘cobweb’ chart with growth and equity prices on separate axis.
In the course of the Long Waves discussion in the 1980s, the topic of eclipses as market influences came up. Arch Crawford argued at that time that a particular eclipse was important due to a concurrent planetary alignment. I am categorically against the idea that planets influence anything. i countered that if eclipses were relevant, those eclipses where the the three bodies (sun, moon, earth) were most perfectly aligned should have the most influence.
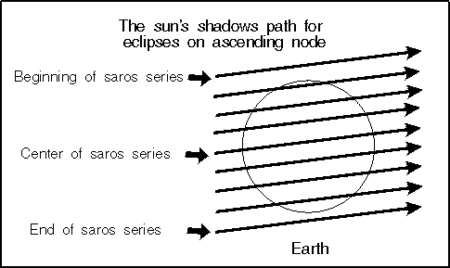
One way to define eclipses is by their ‘saros cycle.’ Eclipses separated by 18 years are very similar. The next illustration shows how solar eclipses of a particular saros cycle progress. The shadow in the early parts of the saros series barely graze the earth. As the series progresses, the shadows get closer to crossing the center of the earth. They then continue to move towards the opposite pole until that particular series moves off of the planet.
click chart to enlarge
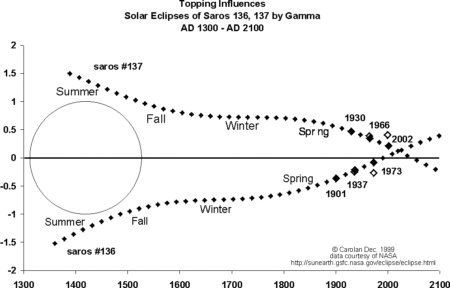
An eclipse can be defined by its “gamma” the value depicting how close to the center of the earth the shadow passes. Eclipses with a gamma of zero are pass the center of the earth. Gamma values of 1 or -1 will be at the poles. Values beyond those will mark partial eclipses.
click chart to enlarge
The chart above plots the gamma values of two solar saros series, #136 and #137, as they progress from their beginning to just beyond their center points. What I noticed in my research was that years containing central eclipses approaching zero gamma correlated with long wave equity peaks.
click chart to enlarge
Here’s a pretty chart showing 2100 years of solar eclipses plotted by their gamma values. There’s an obvious long-term cycle there running over 500 years. The shaded areas are my observation that certain periods of exceptional human accomplishment (the Roman empire, the renaissance, and the 20th century) occurred during similar periods within that long cycle.
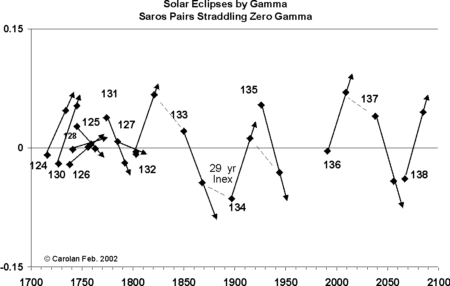
While 18 years separate eclipses in a saros series. Saros series themselves are separated by, on average, twenty-nine years. That period is called an inex. Just as successive eclipses on a saros will have similarities, so too will eclipses on nearby saros. But these sequence of successive saros are not regular. The inex is only an average. So at some periods, these saros are firing off in an orderly manner, but other times not so much, as shown below. My point is don’t expect these cycles to be completely predictable across time. They won’t be.
click chart to enlarge
My hypothesis that emerged from studying long waves and eclipses is that equity price peaks occurred in (or close to) years with solar eclipses near their central, i.e. zero gamma, points. But these tops did not occur at 18 year saros intervals, but rather at every other occurrence, i.e. every 36 years. The 36 year period is very close to the 35.8 years of the 27th Spiral Calendar time period. Some price relationships precisely fit that period, such as the 1937 top to the 1973 top. Now, with multiple saros periods effectively “active” at the same time, there are multiple tops being generated. With successive saros series staggered at 29 year intervals, this timing produced “twin tops” seven years apart. (18 (saros) times 2) minus 29 yr inex = 7 years. I also hypothesized that a similar scheme using lunar eclipses at the center of their saros series correlate with market lows.
The above chart was published in 2002. I published other versions as early as 1999. Extending my hypothesis, my long-wave outlook at that time was for long-wave autumn to end with a “twin tops” in 2002 (late ’01 actually) and 2009, leading to long wave winter that would end with twin lows in 2011 and 2018. The 2018 calculation is based on the lunar saros series that brings this week’s eclipse.
The market did not follow my long waves/eclipse forecast. But what is very interesting is that the expected structure of the cycle has been accurate. Long wave autumn DID end with a pair of twin tops separated by seven years. They just occurred in 2000 and 2007 instead of the expected 2002 and 2009. And the first low of long wave winter did occur, but again it arrived two years early, in 2009 instead of 2011.
An interesting feature of this model is that at times tops and bottoms are forecast quite close together, compressing a major, secular cycle into a very short time frame. The 1973 to late 1974 bear market is one such example. The 1973 top is the second of the twin tops marking the end of long wave spring while the late 1974 low is the first marking the end of long wave summer. The model forecast a similar, compressed and extreme decline for 2009 to 2011. Again, the structure was correct, but the timing was off by two years. An extreme, secular decline did occur from 2007 to 2009.
We are left to wonder. Where is the second low to end long wave winter? Is that slight PE drop in 2016 that low, also two years early than its original 2018 schedule? Is the two year offset a result of the changing of the relative inex relationships across time? Perhaps.
click chart to enlarge
Here’s the important question. What has been, and what will be, the effect of central bank policies on the long wave cycle? Central bank money-printing was expressly utilized to avoid a deflationary collapse, i.e. long wave winter. They succeeded. The PE chart rebounded nicely following 2009. Consider how hard central banks have tried to create inflation. Until recently, they have been spectacularly unsuccessful. The long wave model offers a good explanation. Money printing is fertilizer. Spreading fertilizer on the ground will not grow crops in winter, no matter how much is applied. But what happens to those crops when spring finally occurs?
Here are my thoughts going forward.
- Central banks cannot and have not eliminated cycles. They can suppress cycles, but only delay and postpone effects.
- Long wave “spring” is coming. In fact, it may have started in 2016. While central banks actions make the cycle difficult to ascertain in equity markets, the gold low of late 2015 and the interest rate low of 2016 are perfectly in sync with long wave cycle expectations.
- The central bank actions of extreme money printing may reasonably be expected to shorten the positive effects of long wave spring, and prematurely push economies into long wave summer as inflation quickly overheats.
- The 35 year period from 1981’s interest rate top to 2016’s interest rate low fit its long wave expectations to a “T” (for Treasuries!) It is time to prepare for a multi-decade rise in interest rates as the long waves seasons change.
I don’t necessarily expect any specific market event precisely on this week’s central, lunar eclipse. But we should be alert for any new, dramatic moves that signal a new inflationary environment. Sharp moves up in metals and/or crypto currencies as well as sharp moves down in notes and bonds should all be viewed as indicators that markets are entering a new, cyclic regime that is very much, pro-inflation.







Just a quick question. Do you see any correction (short-term) in equities at it applies to the eclipse? My other wave theorems are looking very corrective right now.
Thanks,
Armand